Sampling |
|
To gain access to revision questions, please sign up and log in.
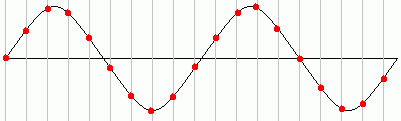
The carrier signal used to carry data or the clock frequency used to sample data needs to be on a frequency at least double the highest data/music/voice frequency.
The Nyquist Frequency also known as the critical frequency is the highest data/music/voice frequency that can be transmitted. This is half the sampling or carrier frequency.
As the transmission frequency increases, the amount of sampled data that can be encoded also increases. For digital transmissions, UHF and Microwave are preferred over the LF, MF and VHF bands. Fibre-optic is even better due to the exceedingly high frequency of light.
The frequency of light is in the region of 1014 Hz. Theoretically data rates of half this figure should be possible if the light emitting and detecting devices and the fibres could ever be made good enough.
Plenty of Samples - This will work - The original signal can be re-constructed fairly well

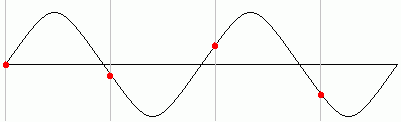
Not Enough Samples - This will not work - The original signal can't be re-constructed
If frequencies above the Nyquist limit or critical frequency are present, these will be unexpected frequencies in the output. These sound unpleasant. A low pass filter fixes the problem and it ensures that no higher frequencies are digitised.
Examples: Poor Sound due to Aliasing COMPARE Better Sound - Much Less Aliasing
If the sampling frequency is too low, unwanted frequencies appear in the re-constructed signal. This problem is known as aliasing. It can often be seen in video recordings where the detail in the recording has a size similar to the pixels in the play-back device. Strange flickering effects can be seen. This is why TV presenters never wear stripy shirts or ties. Other effects include helicopters where the rotor blades appear not to be moving. Here is the video and here is an explanation and here is another and finally.
Here is a still image with aliasing. The camera resolution is similar to the display resolution. To remove the patterns, the camera resolution needs to be at least double that of the display in both the X and Y directions.

Resolution is the size of the smallest step measured in whatever units are appropriate.
Using four bit samples, only 24 = 16 levels are available. This means the signal can not be recreated with great accuracy. The Resolution is poor. Increasing the number of bits per sample improves the resolution. If one extra bit is used in each sample, the resolution doubles. Eight bits per sample give 256 levels and the signal can be reconstructed quite well. The error is one part in 256 which is better than 0.5% error. With 16 bit samples, the error is one part in 65536. This is superbly accurate. CD audio uses this resolution.
reviseOmatic V3 Contacts, ©, Cookies, Data Protection and Disclaimers Hosted at linode.com, London